
We analyse the constraints imposed on the OTO effective theory by microscopic reversibility and thermality of the bath. We determine the additional quartic couplings in this OTO effective action and show their dependence on the bath’s 4-point OTOCs. To overcome this limitation, we construct an extended effective action in a generalised Schwinger-Keldysh framework. The Schwinger-Keldysh effective theory, or the equivalent non-linear Langevin dynamics, is insufficient to determine the out of time order correlators (OTOCs) of the particle. We demonstrate that this quartic effective theory is dual to a stochastic dynamics governed by a non-linear Langevin equation. Working in the Schwinger-Keldysh formalism, we develop an effective action of the particle up to quartic terms. We study the dynamics of a quantum Brownian particle weakly coupled to a thermal bath.

This furnishes a non-trivial check for the validity of these relations derived in the weak coupling regime.A preprint version of the article is available at ArXiv. The local effective theory obeys the recently proposed non-linear fluctuation dissipation theorem relating the non-Gaussianity of thermal noise to the thermal jitter in the damping constant.

Our expression for the influence phase passes non-trivial consistency conditions arising from the underlying unitarity and thermality of the bath. This configuration is the linearised solution of the string motion in the doubled black brane geometry which has been proposed as the holographic dual of a thermal Schwinger-Keldysh contour of the CFT. We compute the Feynman-Vernon influence phase for the heavy quark by evaluating the Nambu-Goto action on a doubled string configuration. In AdS/CFT, this system can be identified with that of a string stretched between the boundary and the horizon of an asymptotically AdS black brane solution. In this work, we consider non-linear corrections to the Langevin effective theory of a heavy quark moving through a strongly coupled CFT plasma. The higher order effective theory has a description in terms of the non-linear Langevin equation with non-Gaussianity in the thermal noise. At quartic order, we explicitly compute the coefficient functions that appear in front of various terms in the effective action in the limit when the background plasma is slowly rotating. We further compute higher order terms in the effective theory of the open scalar field. The noise and dissipation terms in this equation get related by the fluctuation-dissipation relation in presence of chemical potential due to angular momentum.
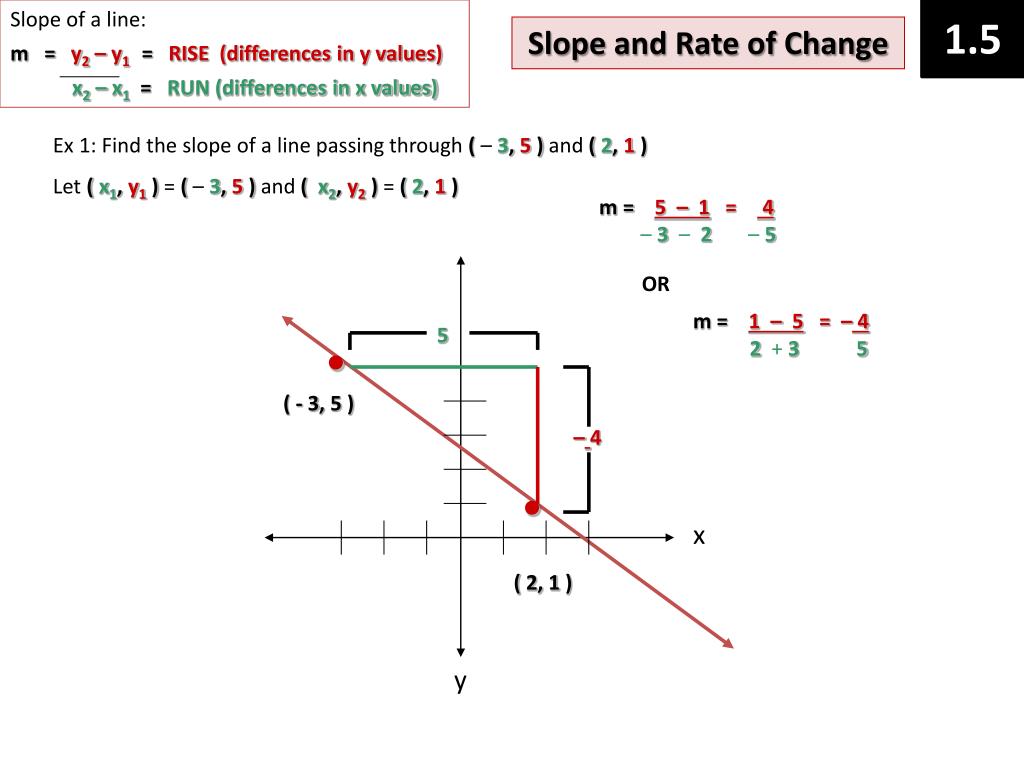
The quadratic effective theory computed using holography leads to the linear Langevin dynamics with rotation. We employ holographic Schwinger-Keldysh path integral techniques to compute the effective theory. The effective theory is determined by the real-time correlation functions of the thermal plasma. For example, the line joining the origin to the point \((a,a)\) makes an angle of 45\(\) as shown in Figure 1.1.We study the effective dynamics of an open scalar field interacting with a strongly-coupled two-dimensional rotating CFT plasma. In a purely mathematical situation, we normally choose the same scale for the \(x\)- and \(y\)-axes. That is, unless stated otherwise, we take “rightward” to be the positive \(x\)-direction and “upward” to be the positive \(y\)-direction. In the \((x,y)\) coordinate system we normally write the \(x\)-axis horizontally, with positive numbers to the right of the origin, and the \(y\)-axis vertically, with positive numbers above the origin. Notation often gets reused and abused in mathematics, but thankfully, it is usually clear from the context what we mean. Previously, we used \((a,b)\) to represent an open interval. In what follows, we use the notation \((x_1,y_1)\) to represent a point in the \((x,y)\) coordinate system, also called the \(x\)-\(y\)-plane.


 0 kommentar(er)
0 kommentar(er)
